Tidal Forces
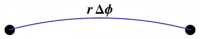
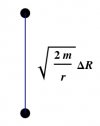
Figure 1: Nearby objects falling radially, either next to each other (at equal
radius), or directly on top of each other.
Consider two nearby objects falling radially. We first consider the case where both objects start at rest on the same $r=\hbox{constant}$ shell, so that they have the same energy $e$. This situation is shown in the first diagram in Figure 1. The separation between the objects is therefore \begin{equation} \Delta s = r \,\Delta\phi \end{equation} and spherical symmetry implies that \begin{equation} \Delta\phi = \hbox{constant} \end{equation} From § Radial Geodesics we know that both trajectories satisfy \begin{equation} \dot{r}^2 = e^2 - \left(1-\frac{2m}{r}\right) \end{equation} which we can differentiate to obtain \begin{equation} \ddot{r} = -\frac{m}{r^2} \label{rddot} \end{equation} since $\dot{r}\ne0$. Thus, \begin{equation} (\Delta s)\Ddot = (r\,\Delta\phi)\Ddot = \ddot{r}\,\Delta\phi = -\frac{m}{r^2} \,\Delta\phi = -\frac{m}{r^3} \,\Delta s \label{tidalphi} \end{equation} As we will show below, the coefficient of $\Delta s$ is a component of curvature. Which one? We are taking two derivatives along the geodesic ($T$-direction) motion of a displacement in the $\phi$-direction, which is changing only in the $\phi$-direction. We claim that \begin{equation} (r\,\Delta\phi\,\phat)\Ddot = -R^\phi{}_{T\phi T}\,\phat \end{equation} which is true since \begin{equation} \Omega^\phi{}_T = \Omega^T{}_\phi = -\frac{m}{r^3} \, \sigma^T \wedge \sigma^\phi \end{equation} (and since $\phat$ does not change in the $r$-direction).
What about two objects falling along the same trajectory, that is, with the same value of $\phi$, but different values of $r$? This situation is shown in the second diagram in Figure 1. According to the metric in rain coordinates, the separation between the objects is now \begin{equation} \Delta s = \sqrt{\frac{2m}{r}}\,\Delta R \end{equation} Assume further that both objects start from rest at infinity, but at different times. Both trajectories therefore correspond to rain observers, for whom the rain coordinate $R$ is constant! Thus, $R$ is constant for each object, and therefore $\Delta R$ remains constant. (This is why rain coordinates are used for this analysis.)
We now have $e=1$, so that \begin{equation} \dot{r} = -\sqrt{\frac{2m}{r}} \end{equation} and \begin{equation} (\Delta s)\Ddot = \left( \sqrt{\frac{2m}{r}}\,\Delta R \right)\DDot = \frac{2m}{r^3} \Delta s \label{tidalR} \end{equation} Since our separation is now measured in the $R$-direction, we should expect \begin{equation} \left(\sqrt{\frac{2m}{r}}\,\Delta R\,\RHAT\right)\DDot = -R^R{}_{TRT}\,\RHAT \end{equation} which is true since \begin{equation} \Omega^R{}_T = \Omega^T{}_R = \frac{2m}{r^3} \, \sigma^T \wedge \sigma^R \end{equation} (and since $\RHAT$ does not change in the $R$-direction).
Notice that falling objects next to each other accelerate toward each other, whereas falling objects above each other accelerate away from each other, just as in the Newtonian case. This shows directly how curvature influences geodesic motion, and supports the interpretation that gravity is curvature.
Equation ($\ref{rddot}$) is in fact identical to the Newtonian expression for the acceleration due to gravity for a point mass; don't forget that we have set $G=1$. Expressions ($\ref{tidalphi}$) and ($\ref{tidalR}$) are therefore formally the same as in Newtonian theory! To see this, note first of all that the argument leading to ($\ref{tidalphi}$) is unchanged, as it relies only on the expression for $\ddot{r}$. As for objects falling on top of each other, the relative acceleration is just the difference of the respective accelerations due to gravity, so that \begin{equation} (\Delta r)\Ddot \approx \frac{d\ddot{r}}{dr} \Delta r = \frac{2m}{r^3} \Delta r \end{equation} Both of these effects are illustrated in Figure 2 for objects falling toward the Earth.


Figure 2: Two objects falling towards the Earth from far away either move closer
together or further apart depending on their initial configuration.
These effects cause tides! To see this, imagine that the Earth is falling towards the Moon, as shown in Figure 3. The Earth itself can be regarded as a rigid body, falling toward the moon with the velocity given by the arrow at its center. But the water on the surface of the Earth falls toward the moon depending on its location, with velocities given by the four arrows shown. The relative motion of the water and the Earth is given by comparing the arrows: Both the nearest and furthest points from the Moon move away from the center, causing high tides, whereas the points on either side move toward the center, causing low tides. This explains why there are two, not one, high (and low) tides every day! A similar effect, but roughly half as strong, is caused by the Sun.
 Figure 3: Tides are caused by the Earth falling towards the Moon!
Figure 3: Tides are caused by the Earth falling towards the Moon!
In both Einstein's theory and Newton's, these results demonstrate the quadrupole nature of gravity; it stretches things in one direction, but shrinks them in the other.