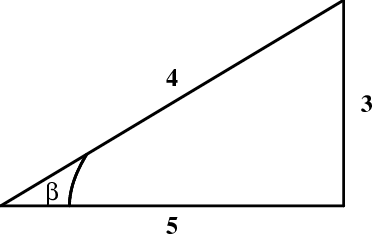
Figure 4.3: A hyperbolic triangle with $\tanh\beta={3\over5}$.

We now recast ordinary triangle trigonometry into hyperbola geometry.

Suppose you know $\tanh\beta={3\over5}$, and you wish to determine $\cosh\beta$. One can of course do this algebraically, using the identity \begin{equation} \cosh^2\beta = {1\over1-\tanh^2\beta} \end{equation} But it is easier to draw any triangle containing an angle whose hyperbolic tangent is $3\over5$. In this case, the obvious choice would be the triangle shown in Figure 4.3 with sides of 3 and 5.

What is $\cosh\beta$? Well, we first need to work out the length $\rho$ of the hypotenuse. The (hyperbolic) Pythagorean Theorem tells us that \begin{equation} 5^2 - 3^2 = \rho^2 \end{equation} so $\rho$ is clearly 4. Take a good look at this 3–4–5 triangle of hyperbola geometry, which is shown in Figure 4.3! But now that we know all the sides of the triangle, it is easy to see that $\cosh\beta={5\over4}$.

Trigonometry is not merely about ratios of sides, it is also about projections. Another common use of triangle trigonometry is to determine the sides of a triangle given the hypotenuse $\rho$ and one angle $\beta$. The answer, of course, is that the sides are $\rho\cosh\beta$ and $\rho\sinh\beta$, as shown in Figure 4.4.

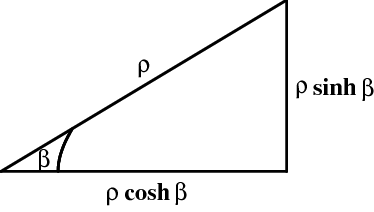Figure 4.4: A hyperbolic triangle in which the hypotenuse and one angle are known.