- §1. The Rindler Metric
- §2. Rindler Geometry
- §3. Rindler Geodesics
- §4. Extending Rindler
Properties of Rindler Geometry
What do Rindler coordinates represent? Consider a worldline with $\rho=\hbox{constant}$, as shown in Figure 1. Since $x^2-t^2=\hbox{constant}$, this worldline is a timelike hyperbola, and is in fact one of our calibrating hyperbolas from special relativity, at constant “distance” $\rho$ from the origin.
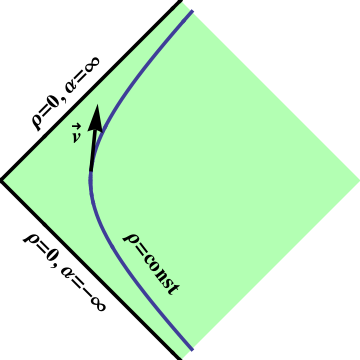 Figure 1: The two-velocity $\vv$ of a Rindler observer, moving along a trajectory
with $\rho$=constant.
Figure 1: The two-velocity $\vv$ of a Rindler observer, moving along a trajectory
with $\rho$=constant.
We claim this worldline is that of an observer undergoing constant acceleration. First of all, we have \begin{equation} v = \frac{dx}{dt} = \frac{dx/d\alpha}{dt/d\alpha} = \tanh\alpha \end{equation} Thus, the parameter $\alpha$ tells us how fast the observer is moving at any point along the trajectory, according to a standard Minkowski observer at rest. Since radial lines $(\alpha=\hbox{constant})$ are orthogonal to such hyperbolas, such lines represent “instants of time” for our accelerating observer. To determine the acceleration, however, it is not enough to differentiate the speed $v$. Rather, one must differentiate the velocity vector, in this case the two-velocity \begin{equation} \vv = \frac{d\rr}{d\tau} \end{equation} as shown in Figure 1. In standard Minkowski coordinates $(t,x)$, we have \begin{equation} d\rr = dt\,\That + dx\,\xhat \end{equation} Furthermore, proper time $\tau$ along the curve is obtained by setting $d\rho=0$ in the line element, so that \begin{equation} d\tau = \rho\,d\alpha \end{equation} Thus, \begin{equation} \vv = \frac{1}{\rho}\frac{d\rr}{d\alpha} \end{equation} which is usually written as \begin{equation} \vv = \begin{pmatrix} \dot{t} \\ \dot{x} \\ \end{pmatrix} = \begin{pmatrix} \cosh\alpha \\ \sinh\alpha \\ \end{pmatrix} \end{equation} which is clearly a unit (timelike) vector, as expected. Differentiating a second time, we obtain \begin{equation} \frac{d\vv}{d\tau} = \frac{1}{\rho}\frac{d\vv}{d\alpha} = \frac{1}{\rho} \begin{pmatrix} \sinh\alpha \\ \cosh\alpha \\ \end{pmatrix} \end{equation} and the magnitude of this vector is clearly constant, namely $1/\rho$.
Thus, $\rho=\hbox{constant}$ curves in the Rindler geometry describe observers undergoing constant acceleration, which however depends on the “radius” $\rho$, and $\alpha=\hbox{constant}$ curves describe surfaces of constant time as seen by these observers. Within the Rindler wedge, $\rho$ runs from $0$ to $\infty$, while $\alpha$ runs from $-\infty$ to $\infty$.